Sorting is a way of arranging items in a systematic manner. Quicksort is the widely used sorting algorithm that makes n log n comparisons in average case for sorting an array of n elements. It is a faster and highly efficient sorting algorithm. This algorithm follows the divide and conquer approach. Divide and conquer is a technique of breaking down the algorithms into subproblems, then solving the subproblems, and combining the results back together to solve the original problem.
Divide: In Divide, first pick a pivot element. After that, partition or rearrange the array into two sub-arrays such that each element in the left sub-array is less than or equal to the pivot element and each element in the right sub-array is larger than the pivot element.
Conquer: Recursively, sort two subarrays with Quicksort.
Combine: Combine the already sorted array.
Quicksort picks an element as pivot, and then it partitions the given array around the picked pivot element. In quick sort, a large array is divided into two arrays in which one holds values that are smaller than the specified value (Pivot), and another array holds the values that are greater than the pivot.
After that, left and right sub-arrays are also partitioned using the same approach. It will continue until the single element remains in the sub-array.
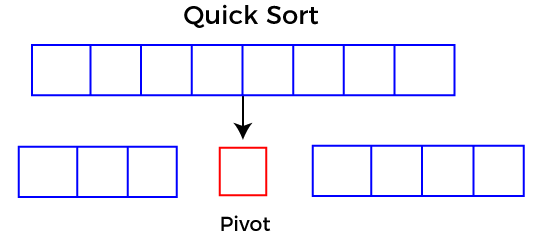
Choosing the pivot
Picking a good pivot is necessary for the fast implementation of quicksort. However, it is typical to determine a good pivot. Some of the ways of choosing a pivot are as follows -
- Pivot can be random, i.e. select the random pivot from the given array.
- Pivot can either be the rightmost element of the leftmost element of the given array.
- Select median as the pivot element.
Quick Sort Pivot Algorithm
Based on our understanding of partitioning in quick sort, we will now try to write an algorithm for it, which is as follows.
Step 1 − Choose the highest/lowest index value has pivotStep 2 − Take two variables to point left and right of the list excluding pivot
Step 3 − left points to the low index
Step 4 − right points to the high
Step 5 − while value at left is less than pivot move right
Step 6 − while value at right is greater than pivot move left
Step 7 − if both step 5 and step 6 does not match swap left and right
Step 8 − if left ≥ right, the point where they met is new pivot
Algorithm

Working of Quick Sort Algorithm
Now, let's see the working of the Quicksort Algorithm.
To understand the working of quick sort, let's take an unsorted array. It will make the concept more clear and understandable.
Let the elements of array are -

In the given array, we consider the leftmost element as pivot. So, in this case, a[left] = 24, a[right] = 27 and a[pivot] = 24.
Since, pivot is at left, so algorithm starts from right and move towards left.
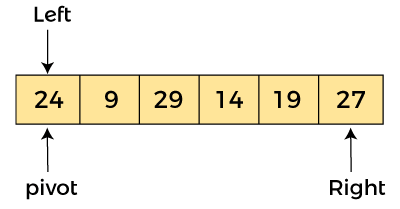
Now, a[pivot] < a[right], so algorithm moves forward one position towards left, i.e. -
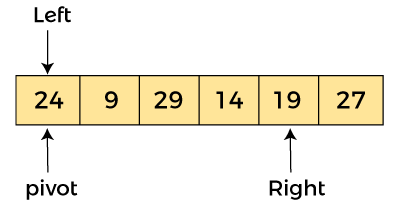
Now, a[left] = 24, a[right] = 19, and a[pivot] = 24.
Because, a[pivot] > a[right], so, algorithm will swap a[pivot] with a[right], and pivot moves to right, as -
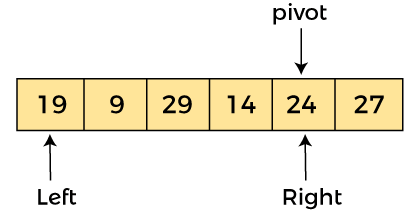
Now, a[left] = 19, a[right] = 24, and a[pivot] = 24. Since, pivot is at right, so algorithm starts from left and moves to right.
As a[pivot] > a[left], so algorithm moves one position to right as -
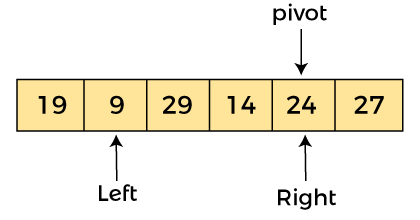
Now, a[left] = 9, a[right] = 24, and a[pivot] = 24. As a[pivot] > a[left], so algorithm moves one position to right as -
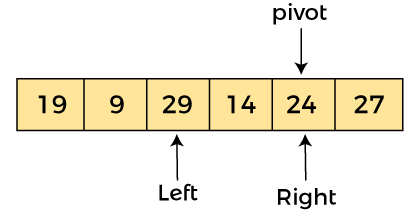
Now, a[left] = 29, a[right] = 24, and a[pivot] = 24. As a[pivot] < a[left], so, swap a[pivot] and a[left], now pivot is at left, i.e. -
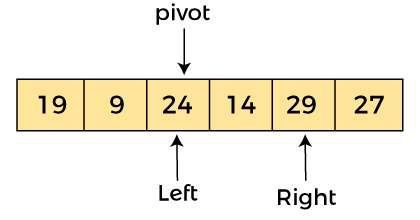
Since, pivot is at left, so algorithm starts from right, and move to left. Now, a[left] = 24, a[right] = 29, and a[pivot] = 24. As a[pivot] < a[right], so algorithm moves one position to left, as -

Now, a[pivot] = 24, a[left] = 24, and a[right] = 14. As a[pivot] > a[right], so, swap a[pivot] and a[right], now pivot is at right, i.e. -
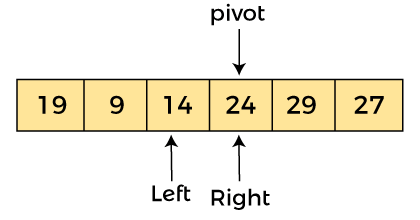
Now, a[pivot] = 24, a[left] = 14, and a[right] = 24. Pivot is at right, so the algorithm starts from left and move to right.
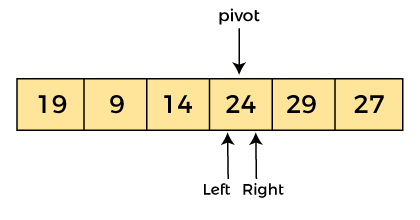
Now, a[pivot] = 24, a[left] = 24, and a[right] = 24. So, pivot, left and right are pointing the same element. It represents the termination of procedure.
Element 24, which is the pivot element is placed at its exact position.
Elements that are right side of element 24 are greater than it, and the elements that are left side of element 24 are smaller than it.
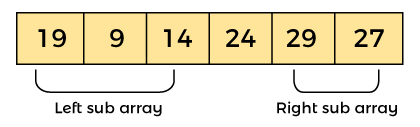
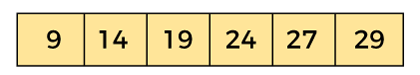
Now, in a similar manner, quick sort algorithm is separately applied to the left and right sub-arrays. After sorting gets done, the array will be -
Example
Example of Non Recursive Quick Sort:
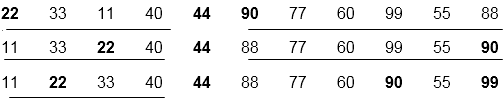
1. 44 33 11 55 77 90 40 60 99 22 88
Let 44 be the Pivot element
and scanning done from right to left
Comparing 44 to the
right-side elements, and if right-side elements are smaller than 44,
then swap it. As 22 is smaller than 44 so
swap them.
22 33 11 55 77 90 40 60 99 44 88
Now comparing 44 to the left
side element and the element must be greater than 44 then swap
them. As 55 are greater than 44 so swap them.
22 33 11 44 77 90 40 60 99 55 88
Recursively, repeating steps 1 & steps 2
until we get two lists one left from pivot element 44 &
one right from pivot element.
22 33 11 40 77 90 44 60 99 55 88
Swap with 77:
22 33 11 40 44 90 77 60 99 55 88
Now, the element on the right side and left side are greater than and smaller than 44 respectively.
Now we get two sorted lists:
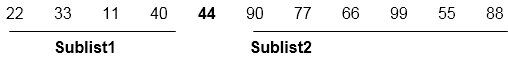
And these sublists are sorted under the same process as above done.
These two sorted sublists side by side.
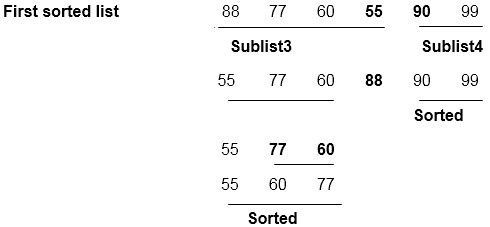
Now we get two sorted lists:
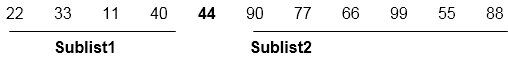
And these sublists are sorted under the same process as above done.
These two sorted sublists side by side.


Merging Sublists:
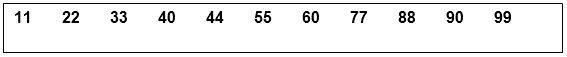
SORTED LISTS

SORTED LISTS
Quick-sort complexity
Now, let's see the time complexity of quicksort in best case, average case, and in worst case. We will also see the space complexity of quicksort.
1. Time Complexity
|
Case |
Time Complexity |
|
Best
Case |
O(n*logn) |
|
Average
Case |
O(n*logn) |
|
Worst
Case |
O(n2) |
- Best Case Complexity - In Quicksort, the best-case occurs when the pivot element is the middle element or near to the middle element. The best-case time complexity of quicksort is O(n*logn).
- Average Case Complexity - It occurs when the array elements are in jumbled order that is not properly ascending and not properly descending. The average case time complexity of quicksort is O(n*logn).
- Worst Case Complexity - In quick sort, worst case occurs when the pivot element is either greatest or smallest element. Suppose, if the pivot element is always the last element of the array, the worst case would occur when the given array is sorted already in ascending or descending order. The worst-case time complexity of quicksort is O(n2).
Though the worst-case complexity of quicksort is more than other sorting algorithms such as Merge sort and Heap sort, still it is faster in practice. Worst case in quick sort rarely occurs because by changing the choice of pivot, it can be implemented in different ways. Worst case in quicksort can be avoided by choosing the right pivot element.
2. Space Complexity
|
Space
Complexity |
O(n*logn) |
|
Stable |
NO |
- The space complexity of quicksort is O(n*logn).
Implementation of quicksort
Now, let's see the programs of quicksort in different programming languages.
Program: Write a program to implement quicksort in C language.
#include <stdio.h>
/* function that consider last element as pivot,
place the pivot at its exact position, and place
smaller elements to left of pivot and greater
elements to right of pivot. */
int partition (int a[], int start, int end)
{
int pivot = a[end]; // pivot element
int i = (start - 1);
for (int j = start; j <= end - 1; j++)
{
// If current element is smaller than the pivot
if (a[j] < pivot)
{
i++; // increment index of smaller element
int t = a[i];
a[i] = a[j];
a[j] = t;
}
}
int t = a[i+1];
a[i+1] = a[end];
a[end] = t;
return (i + 1);
}
/* function to implement quick sort */
void quick(int a[], int start, int end) /* a[] = array to be sorted, start = Starting index, end = Ending index */
{
if (start < end)
{
int p = partition(a, start, end); //p is the partitioning index
quick(a, start, p - 1);
quick(a, p + 1, end);
}
}
/* function to print an array */
void printArr(int a[], int n)
{
int i;
for (i = 0; i < n; i++)
printf("%d ", a[i]);
}
int main()
{
int a[] = { 24, 9, 29, 14, 19, 27 };
int n = sizeof(a) / sizeof(a[0]);
printf("Before sorting array elements are - \n");
printArr(a, n);
quick(a, 0, n - 1);
printf("\nAfter sorting array elements are - \n");
printArr(a, n);
return 0;
}
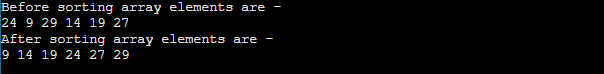






No comments:
Post a Comment